Sistemas de Coordenadas¶

Visão Global¶

Projecções cartográficas tentam representar a superfície da terra ou uma parte da terra num pedaço de papel ou ecrã de computador. Um sistema de coordenadas (SC) define, por isso, com a ajuda de coordenadas, como o mapa projectado pelo seu SIG, bi-dimensional, se relaciona com os locais reais na terra. A decisão sobre qual a projecção cartográfica e qual o sistema de coordenadas usar, depende da extensão e região da área onde deseja trabalhar, da análise que deseja realizar, e, frequentemente, da disponibilidade de dados.

Projecção Cartográfica em detalhe¶

Um método tradicional de representar a forma da terra é usar esferas. Há, no entanto, um problema com esta abordagem. Embora esferas preservem a maioria da forma da terra e ilustrem a configuração espacial de elementos de dimensão continental, são difíceis de carregar num bolso. São também apenas convenientes de usar em escalas extremamente pequenas (p. ex. 1:100 milhões).

A maioria dos dados de mapas temáticos utilizados em aplicações SIG têm uma escala consideravelmente maior. Conjuntos de dados SIG típicos têm escalas de 1:250 000 ou maiores, dependendo do nível de detalhe. Uma esfera com este tamanho seria difícil e dispendioso de produzir e ainda mais difícil de transportar. Consequentemente, os cartógrafos desenvolveram um conjunto de técnicas designadas por projecções cartográficas concebidas para representar, com precisão razoável, a terra esférica em duas dimensões.

Quando olhada de perto a terra aparenta ser relativamente plana. Contudo quando olhada a partir do espaço, podemos ver que a terra é relativamente esférica. Mapas, como aqueles que veremos posteriormente no tópico dedicado à produção de mapas, são representações da realidade. São concebidos para não apenas representar entidades, mas também a sua forma e disposição espacial. Cada projecção cartográfica tem vantagens e desvantagens. A melhor projecção para um mapa depende da escala do mapa, e dos objectivos para os quais será usado. Por exemplo, uma projecção poderá ter distorções inaceitáveis se usada num mapa de todo o continente Africano, mas poderá ser uma excelente escolha para um mapa numa escala grande (detalhado) do seu país. As propriedades de uma projecção cartográfica podem também influenciar algumas características na concepção do mapa. Algumas projecções são indicadas para pequenas áreas, outras são indicadas para representar áreas com uma grande extensão Este-Oeste, e outras são mais apropriadas para representar áreas com uma grande extensão Norte-Sul.

As três famílias das projecções cartográficas¶

O processo de criar projecções cartográficas pode ser visualizado posicionando uma fonte de luz dentro de um globo transparente no qual estão colocadas entidades opacas. Então projectam-se as delimitações dessas entidades num papel plano bidimensional. Diferentes formas de projectar podem ser criadas ao rodear o globo com o papel num ajuste cilindrico, ou cónico, ou mesmo como uma superfície plana. Cada um destes métodos produz o que se designa de um tipo de projecção cartográfica. Desta forma, há um tipo de projecção planar, um tipo de projecção cilíndrica, e outro tipo denominado de projecção cónica (ver figure_projections_families)

Figure Projection Families 1:

Os três tipos de projecções cartográficas. Podem ser representadas por a) projecções cilíndricas, b) projecções cónicas ou c) projecções planares.

Hoje, naturalmente, o processo de projectar uma terra esférica num papel plano é feito usando princípios matemáticos de geometria e trigonometria, reproduzindo-se a projecção física de luz através do globo.

Precisão das projecções cartográficas¶

Projecções cartográficas nunca são representações totalmente precisas da terra esférica. Como resultado do processo de projecção, cada mapa mostra distorções de ângulo, distância e área. Uma projecção pode combinar várias destas características ou pode ser um compromisso que distorce todas as propriedades de área, distância, e conformidade angular, dentro de alguns limites aceitáveis. Exemplos de projecções com este tipo de compromissos são a projecção de Winkel Tripel e a projecção de Robinson (ver figure_robinson_projection), que são muitas vezes usadas em mapas do mundo.

Figure Robinson Projection 1:

A projecção de Robinson é um compromisso entre distorções de área, ângulo e direcção, e distância que são aceitáveis.

É geralmente impossível preservar todas as características em simultâneo numa projecção cartográfica. Isto significa que quando queremos executar operações analíticas precisas necessitamos de usar uma projecção cartográfica que fornece as melhores características para as nossas análises. Por exemplo, se for necessário medir distâncias no nosso mapa, devemos usar uma projecção que garante uma elevada precisão nas distâncias.

Projecções cartográficas com conformidade angular¶

Ao trabalhar com um globo, as principais direcções da rosa dos ventos (Norte, Este, Sul e Oeste) ocorrerão sempre a 90º umas das outras. Por outras palavras, Este ocorrerá sempre num ângulo de 90º com a direcção Norte. As propriedades angulares correctas podem ser preservadas numa projecção. Uma projecção que mantém ângulos e direcções é designada de projecção conforme ou projecção ortomórfica.

Estas projecções são usadas quando a preservação das relações angulares é importante. São usadas geralmente para tarefas de navegação ou meteorologia. É importante relembrar que manter verdadeiros os ângulos num mapa é difícil para grandes áreas e deve ser apenas tentado para pequenas porções da terra. O tipo de projecções Conformes resultam em distorções de áreas, o que significa que se forem efectuadas medições de área no mapa, estas serão incorrectas. Quanto maior a área menos precisas serão as medições de área. Exemplos são a projecção de Mercator (como ilustrado na figure_mercator_projection) e a projecção Cónica Conforme de Lambert. O U.S. Geological Survey usa uma projecção conforme para muitros dos seus mapas topográficos.

Figure Mercator Projection 1:

The Mercator projection, for example, is used where angular relationships are important, but the relationship of areas are distorted.

Map projections with equal distance¶

If your goal in projecting a map is to accurately measure distances, you should select a projection that is designed to preserve distances well. Such projections, called equidistant projections, require that the scale of the map is kept constant. A map is equidistant when it correctly represents distances from the centre of the projection to any other place on the map. Equidistant projections maintain accurate distances from the centre of the projection or along given lines. These projections are used for radio and seismic mapping, and for navigation. The Plate Carree Equidistant Cylindrical (see figure_plate_caree_projection) and the Equirectangular projection are two good examples of equidistant projections. The Azimuthal Equidistant projection is the projection used for the emblem of the United Nations (see figure_azimuthal_equidistant_projection).

Figure Plate Carree Projection 1:

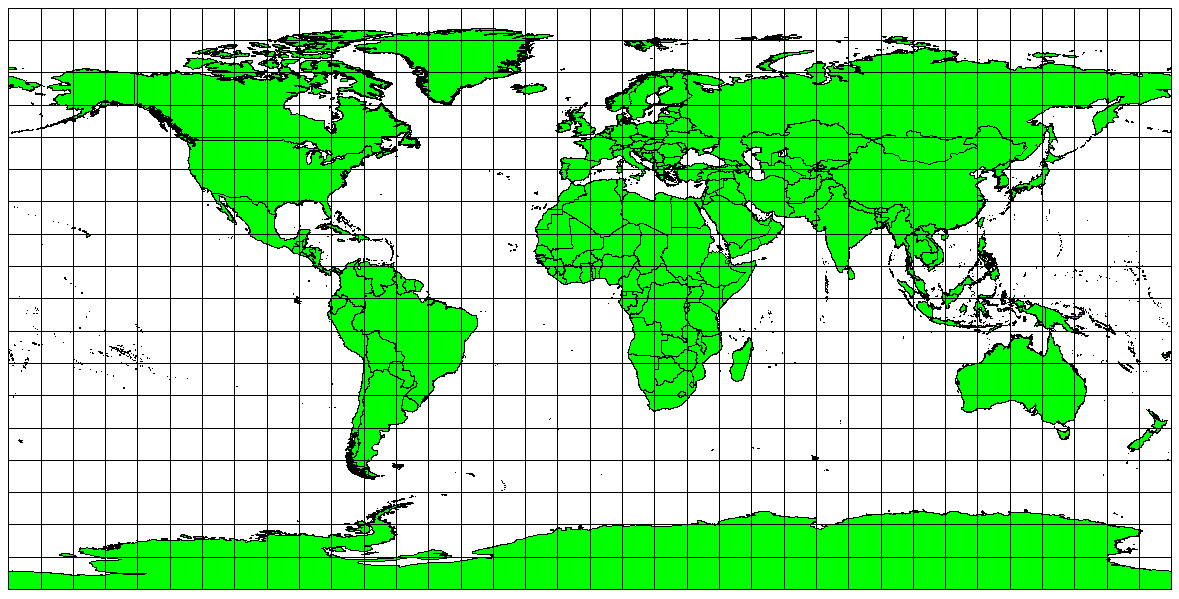
A projecção Cilíndrica Equidistante de Plate Carree, por exemplo, é usada quando a medição precisa de distâncias é importante.

Figure Azimuthal Equidistant Projection 1:

O Logo das Nações Unidas usa a projecção Equidistante Azimutal.

Projecções com áreas equivalentes¶

Quando um mapa representa áreas sobre todo o mapa de forma a que todas as áreas cartografadas têm a mesma relação proporcional com as áreas na Terra que representam, então o mapa é um mapa de áreas equivalentes. Na prática, mapas de referência geral e mapas educacionais necessitam frequentemente de utilizar projecções equivalentes. Como o nome indica, estes mapas são especialmente úteis quando o cálculo de áreas é o uso dominante que lhes é dado. Se, por exemplo, tiver necessidade de analisar uma área particular na sua cidade para descobrir se é suficientemente grande para um novo centro comercial, então as projecções equivalentes são a melhor escolha. Por outro lado, quanto maior a área em análise mais precisas serão as medições de área que fizer, se usar uma projecção equivalente em vez de qualquer outro tipo de projecção. Por outro lado, uma projecção equivalente resulta na distorção da conformidade angular ao lidar com áreas grandes. Pequenas áreas serão muito menos sujeitas a verem os seus ângulos distorcidos ao usar uma projecção equivalente. As projecções Cilíndricas Equivalentes de Alber, de Lambert, e de Mollweide (representadas na figure_mollweide_equal_area_projection) são exemplos de projecções equivalentes muito usadas em trabalho com SIG.

Figure Mollweide Equal Area Projection 1:

A projecção Cilíndrica Equivalente de Mollweide, por exemplo, garante que todas as áreas cartografas têm a mesma relação proporcional com as áreas na Terra.

Tenha em atenção que as projecções cartográficas são um tópico muito complexo. Existem centenas de diferentes projecções disponíveis em todo o mundo, cada tentando retratar uma certa porção da superfície da terra o mais fielmente possível num pedaço plano de papel. Na realidade, a escolha de qual a projecção a usar será frequentemente estará já tomada. A maioria dos países têm as suas projecções mais comuns e quando informação é trocada, em geral segue-se a norma nacional.

Sistemas de Coordenadas (SC) em detalhe¶

With the help of coordinate reference systems (CRS) every place on the earth can be specified by a set of three numbers, called coordinates. In general CRS can be divided into projected coordinate reference systems (also called Cartesian or rectangular coordinate reference systems) and geographic coordinate reference systems.

Geographic Coordinate Systems¶

The use of Geographic Coordinate Reference Systems is very common. They use degrees of latitude and longitude and sometimes also a height value to describe a location on the earth’s surface. The most popular is called WGS 84.

Lines of latitude run parallel to the equator and divide the earth into 180 equally spaced sections from North to South (or South to North). The reference line for latitude is the equator and each hemisphere is divided into ninety sections, each representing one degree of latitude. In the northern hemisphere, degrees of latitude are measured from zero at the equator to ninety at the north pole. In the southern hemisphere, degrees of latitude are measured from zero at the equator to ninety degrees at the south pole. To simplify the digitisation of maps, degrees of latitude in the southern hemisphere are often assigned negative values (0 to -90°). Wherever you are on the earth’s surface, the distance between the lines of latitude is the same (60 nautical miles). See figure_geographic_crs for a pictorial view.

Figure Geographic CRS 1:

Geographic coordinate system with lines of latitude parallel to the equator and lines of longitude with the prime meridian through Greenwich.

Lines of longitude, on the other hand, do not stand up so well to the standard of uniformity. Lines of longitude run perpendicular to the equator and converge at the poles. The reference line for longitude (the prime meridian) runs from the North pole to the South pole through Greenwich, England. Subsequent lines of longitude are measured from zero to 180 degrees East or West of the prime meridian. Note that values West of the prime meridian are assigned negative values for use in digital mapping applications. See figure_geographic_crs for a pictorial view.

At the equator, and only at the equator, the distance represented by one line of longitude is equal to the distance represented by one degree of latitude. As you move towards the poles, the distance between lines of longitude becomes progressively less, until, at the exact location of the pole, all 360° of longitude are represented by a single point that you could put your finger on (you probably would want to wear gloves though). Using the geographic coordinate system, we have a grid of lines dividing the earth into squares that cover approximately 12363.365 square kilometres at the equator — a good start, but not very useful for determining the location of anything within that square.

To be truly useful, a map grid must be divided into small enough sections so that they can be used to describe (with an acceptable level of accuracy) the location of a point on the map. To accomplish this, degrees are divided into minutes (') and seconds ("). There are sixty minutes in a degree, and sixty seconds in a minute (3600 seconds in a degree). So, at the equator, one second of latitude or longitude = 30.87624 meters.

Projected coordinate reference systems¶

A two-dimensional coordinate reference system is commonly defined by two axes. At right angles to each other, they form a so called XY-plane (see figure_projected_crs on the left side). The horizontal axis is normally labelled X, and the vertical axis is normally labelled Y. In a three-dimensional coordinate reference system, another axis, normally labelled Z, is added. It is also at right angles to the X and Y axes. The Z axis provides the third dimension of space (see figure_projected_crs on the right side). Every point that is expressed in spherical coordinates can be expressed as an X Y Z coordinate.

Figure Projected CRS 1:

Two and three dimensional coordinate reference systems.

A projected coordinate reference system in the southern hemisphere (south of the equator) normally has its origin on the equator at a specific Longitude. This means that the Y-values increase southwards and the X-values increase to the West. In the northern hemisphere (north of the equator) the origin is also the equator at a specific Longitude. However, now the Y-values increase northwards and the X-values increase to the East. In the following section, we describe a projected coordinate reference system, called Universal Transverse Mercator (UTM) often used for South Africa.

Universal Transverse Mercator (UTM) CRS in detail¶

The Universal Transverse Mercator (UTM) coordinate reference system has its origin on the equator at a specific Longitude. Now the Y-values increase southwards and the X-values increase to the West. The UTM CRS is a global map projection. This means, it is generally used all over the world. But as already described in the section ‘accuracy of map projections’ above, the larger the area (for example South Africa) the more distortion of angular conformity, distance and area occur. To avoid too much distortion, the world is divided into 60 equal zones that are all 6 degrees wide in longitude from East to West. The UTM zones are numbered 1 to 60, starting at the international date line (zone 1 at 180 degrees West longitude) and progressing East back to the international date line (zone 60 at 180 degrees East longitude) as shown in figure_utm_zones.

Figure UTM Zones 1:

The Universal Transverse Mercator zones. For South Africa UTM zones 33S, 34S, 35S, and 36S are used.

As you can see in figure_utm_zones and figure_utm_for_sa, South Africa is covered by four UTM zones to minimize distortion. The zones are called UTM 33S, UTM 34S, UTM 35S and UTM 36S. The S after the zone means that the UTM zones are located south of the equator.

Figure UTM for South Africa 1:

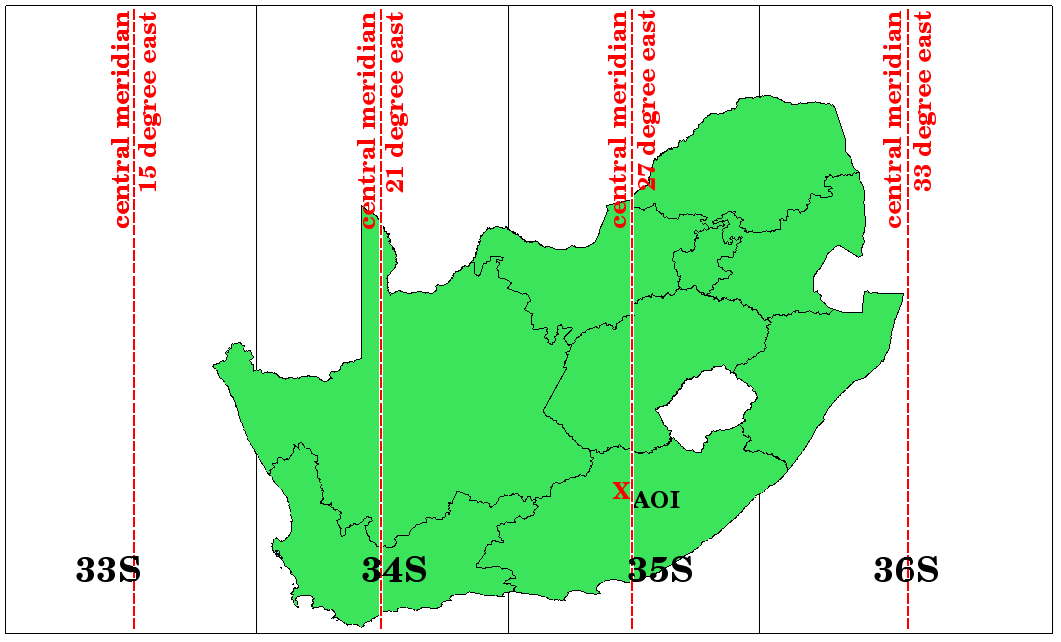
UTM zones 33S, 34S, 35S, and 36S with their central longitudes (meridians) used to project South Africa with high accuracy. The red cross shows an Area of Interest (AOI).

Say, for example, that we want to define a two-dimensional coordinate within the Area of Interest (AOI) marked with a red cross in figure_utm_for_sa. You can see, that the area is located within the UTM zone 35S. This means, to minimize distortion and to get accurate analysis results, we should use UTM zone 35S as the coordinate reference system.

The position of a coordinate in UTM south of the equator must be indicated with the zone number (35) and with its northing (y) value and easting (x) value in meters. The northing value is the distance of the position from the equator in meters. The easting value is the distance from the central meridian (longitude) of the used UTM zone. For UTM zone 35S it is 27 degrees East as shown in figure_utm_for_sa. Furthermore, because we are south of the equator and negative values are not allowed in the UTM coordinate reference system, we have to add a so called false northing value of 10,000,000 m to the northing (y) value and a false easting value of 500,000 m to the easting (x) value. This sounds difficult, so, we will do an example that shows you how to find the correct UTM 35S coordinate for the Area of Interest.

On-The-Fly Projection¶

As you can probably imagine, there might be a situation where the data you want to use in a GIS are projected in different coordinate reference systems. For example, you might get a vector layer showing the boundaries of South Africa projected in UTM 35S and another vector layer with point information about rainfall provided in the geographic coordinate system WGS 84. In GIS these two vector layers are placed in totally different areas of the map window, because they have different projections.

To solve this problem, many GIS include a functionality called оn-the-fly projection. It means, that you can define a certain projection when you start the GIS and all layers that you then load, no matter what coordinate reference system they have, will be automatically displayed in the projection you defined. This functionality allows you to overlay layers within the map window of your GIS, even though they may be in different reference systems.

Common problems / things to be aware of¶

The topic map projection is very complex and even professionals who have studied geography, geodetics or any other GIS related science, often have problems with the correct definition of map projections and coordinate reference systems. Usually when you work with GIS, you already have projected data to start with. In most cases these data will be projected in a certain CRS, so you don’t have to create a new CRS or even re project the data from one CRS to another. That said, it is always useful to have an idea about what map projection and CRS means.

What have we learned?¶

Let’s wrap up what we covered in this worksheet:

• Map projections portray the surface of the earth on a two-dimensional, flat piece of paper or computer screen.
• There are global map projections, but most map projections are created and optimized to project smaller areas of the earth’s surface.
• Map projections are never absolutely accurate representations of the spherical earth. They show distortions of angular conformity, distance and area. It is impossible to preserve all these characteristics at the same time in a map projection.
• A Coordinate reference system (CRS) defines, with the help of coordinates, how the two-dimensional, projected map is related to real locations on the earth.
• There are two different types of coordinate reference systems: Geographic Coordinate Systems and Projected Coordinate Systems.
• On the Fly projection is a functionality in GIS that allows us to overlay layers, even if they are projected in different coordinate reference systems.

Now you try!¶

Here are some ideas for you to try with your learners:

• Start QGIS and load two layers of the same area but with different projections and let your pupils find the coordinates of several places on the two layers. You can show them that it is not possible to overlay the two layers. Then define the coordinate reference system as Geographic/WGS 84 inside the Project Properties dialog and activate the checkbox Enable on-the-fly CRS transformation. Load the two layers of the same area again and let your pupils see how on-the-fly projection works.
• You can open the Project Properties dialog in QGIS and show your pupils the many different Coordinate Reference Systems so they get an idea of the complexity of this topic. With ‘on-the-fly’ CRS transformation enabled you can select different CRS to display the same layer in different projections.

Something to think about¶

If you don’t have a computer available, you can show your pupils the principles of the three map projection families. Get a globe and paper and demonstrate how cylindrical, conical and planar projections work in general. With the help of a transparency sheet you can draw a two-dimensional coordinate reference system showing X axes and Y axes. Then, let your pupils define coordinates (x and y values) for different places.

Further reading¶

Books:

• Chang, Kang-Tsung (2006). Introduction to Geographic Information Systems. 3rd Edition. McGraw Hill. ISBN: 0070658986
• DeMers, Michael N. (2005). Fundamentals of Geographic Information Systems. 3rd Edition. Wiley. ISBN: 9814126195
• Galati, Stephen R. (2006): Geographic Information Systems Demystified. Artech House Inc. ISBN: 158053533X

Websites:

• http://www.colorado.edu/geography/gcraft/notes/mapproj/mapproj_f.html
• http://geology.isu.edu/geostac/Field_Exercise/topomaps/index.htm

The QGIS User Guide also has more detailed information on working with map projections in QGIS.

What’s next?¶

In the section that follows we will take a closer look at Map Production.